BODMAS Questions

Mathematics can often seem overwhelming, especially when dealing with complex expressions that involve multiple operations. The BODMAS rule is an essential concept that helps break down these challenges by explaining the correct order of operations in mathematical expressions. It is widely used to simplify equations and reduce calculation time, making it crucial to master this concept from the outset.

Whether you’re a student learning math or preparing for competitive exams like SAT, GMAT, or GRE, having a solid grasp of BODMAS is critical to solving problems accurately and efficiently. This blog provides a comprehensive guide to BODMAS questions, offering valuable practice to help you build a solid foundation in this essential mathematical skill.

What is BODMAS?

BODMAS is an acronym that stands for:

B: Brackets
O: Order of Indices
D: Division
M: Multiplication
A: Addition
S: Subtraction

It’s crucial to follow this order to ensure that you solve expressions correctly. For example, without the BODMAS rule, you might solve expressions out of sequence, leading to incorrect results. Now, let us understand the order in which it is used in mathematics-

B ➝ O ➝ D ➝ M ➝ A ➝ S

Starting from ‘B’ and moving towards the right of the word will give you correct and quick answers for those complex equations. Refer to the table below for a better understanding of this concept.

Letters	Steps
Brackets (B)	Break down the problems inside the brackets
Orders of Indices (O)	Solve the indices such as roots, powers, etc
Division (D)	Divide the numbers which are given
Multiplication (M)	Multiply the numbers next.
Addition (A)	Sum up the next numbers
Subtraction (S)	Subtract the numbers left in the end.

Importance of the BODMAS Rule

The BODMAS rule helps standardize calculations by providing a clear order of operations. If we didn’t follow this rule, people might interpret mathematical problems differently, leading to inconsistent results. This rule applies to all types of mathematical expressions, from basic arithmetic to advanced algebraic equations. It’s used universally in schools, colleges, and in industries like engineering, finance, and computing.

Following BODMAS helps you:

• Prevent errors in calculations
• Ensure consistency in solving expressions
• Improve your overall math skills

Understanding the Components of BODMAS

Let’s break down the components of BODMAS:

1 Brackets

Brackets indicate that certain operations should be performed first. There are different types of brackets:

• Parentheses ( ): These are the most common brackets used in expressions.
• Square brackets [ ]: Used for nested operations.
• Curly braces { }: Less commonly used but still part of the BODMAS rule.

2 Orders (Exponents)

Orders refer to operations involving powers and square roots. This includes:

• Exponents: Squaring a number (e.g., 2²=4) or cubing a number (e.g., 3³=27).
• Roots: Square roots or cube roots (e.g., √9=3).

3 Division and Multiplication

Division and multiplication have the same precedence and should be performed from left to right. For example:

• First, solve the division (÷, /) and then multiplication (×, *) if both appear in the expression.

4 Addition and Subtraction

Addition (+) and subtraction (–) also have the same precedence and should be solved from left to right, just like multiplication and division.

We should perform these actions in the correct order when implementing the BODMAS rule. The table below shows the symbol for each component of BODMAS. Please take a look, as these will be used further.

B	Brackets	( ), { }, [ ]
O	Order of	Square roots, indices, exponents and powers
D	Division	÷, /
M	Multiplication	×, *
A	Addition	+
S	Subtraction	–

Tips to Remember BODMAS Rule

Following are the guidelines for utilising the BODMAS rule to simplify the expression:

• First, make the brackets simpler.
• Exponent or root-term solutions
• Make a division or multiplication (from left to right)
• Carry out the operation of addition or subtraction (from left to right)

BODMAS vs PEMDAS

Two acronyms that are used to recall the order of operations are BODMAS and PEMDAS. Nearly identical to the PEMDAS rule is the BODMAS rule. Because certain terms are recognised by different names in several nations, there is a variance in the abbreviation. The table below makes the distinction between BODMAS and PEMDAS clearer while emphasizing the importance of following the left-to-right rule for division, multiplication, addition, and subtraction in both systems.

Aspect	BODMAS Rule	PEMDAS Rule
Acronym	BODMAS (Brackets, Orders, Division/Multiplication, Addition/Subtraction)	PEMDAS (Parentheses, Exponents, Multiplication/Division, Addition/Subtraction)
Difference in Terminology	“Brackets” and “Orders”	“Parentheses” and “Exponents”
Order of Operations	Brackets, Orders (Exponents), Division, Multiplication, Addition, Subtraction	Parentheses, Exponents, Multiplication, Division, Addition, Subtraction
Division/Multiplication	Solve from left to right when both division and multiplication appear	Solve from left to right when both division and multiplication appear
Addition/Subtraction	Solve from left to right when both addition and subtraction appear	Solve from left to right when both addition and subtraction appear

When to Use BODMAS?

When a mathematical equation has many operations, BODMAS is utilised. The BODMAS approach has a set of guidelines that must be followed in order. This provides a suitable framework to generate an original response for each mathematical phrase.

Conditions to follow:

• If there is any bracket, open the bracket, then add or subtract the terms. a + (b + c) = a + b + c, a + (b – c) = a + b – c
• If there is a negative sign just open the bracket, and multiply the negative sign with each term inside the bracket. a – (b + c) ⇒ a – b – c
• If there is any term just outside the bracket, multiply that outside term with each term inside the bracket. a(b + c) ⇒ ab + ac

BODMAS Quiz

Here are some BODMAS questions for you:

1. Simplify 25 – [20 – {10 -(7-5-3)}
2. Find out the answer for 100 – 3 [20 + {50 – 40}]
3. Solv: 7 + (8 -3×2)
4. What would be the answer for 50- [20 +{ 30- ( 20- 5)}]
5. Find the value of 150- [10 +{ 3- ( 20- 5)}]
6. Simplify 1៖ 3/7 x (6+8X3-2)+ [1/5៖ 7/25 – {3/7 + 8/14} ]
7. Using the rule of BODMAS, determine the answer of 18 ៖ 10 – 4 + 32 ៖ (4+ 10 ៖ 2 – 1)
8. 10 – [ 6 -{7 – (6- 8 – 5)}] solve the following
9. What will the answer of this question 5x ¼ ៖ 3/7 + [45/24- 2/3 + 5/6 x 2/5 ]
10. 1800 ៖ 10 {( 12 – 6) + (24 – 12)}
11. 1/2 [{ -2 (1 + 2) 10} 15] x 3
12. 20 – [6- {4 – (8 – 6 + 3)}]
13. According to the BODMAS rule, find out the value of y: 36 ៖ 2 + y x 3 – 22 = 8
14. Determine the correct answer for- (1/4 + 7/4) – 2
15. 45 x 3 x 7 x [22/11+ 36/12]
16. Solve this question using the BODMAS rule: 2 [2 + 2 {39 -2 (17 + 2)}]
17. Solve this BODMAS Question (17 x 18)៖ 10 x 2 (2+ 13)- 25
18. (3 + 3) x (3 ៖ 3) x (3×3) solve this problem using BODMAS rule
19. 2550 – [510 {270 – ( 90- 80 + 70)}]
20. [29- (- 2) {6 – (7 – 3)}] ៖ [3 x {5 + (-3) x (-2)}] solve this complex equation using the BODMAS rule
21. 63- (-3) {-2 -8-3} ៖ 3 {5+ (-2) (-1)}
22. What will be the answer of this BODMAS question: 27 – [38 – {46 – (15- 13 2)}]
23. 25- 1/25 {5+ 4 – (3+ 2- 1 + 3)}
24. Solve: (6+4)×3−5²

How to Solve BODMAS Questions?

The BODMAS rules simplify a mathematical equation by breaking it down. Let’s solve a BODMAS question to understand its rules.

Example: 4(10+15÷5×4-2×2)

Step 1: Solve the Brackets
Here, you must calculate the inside bracket first.
4(10+15÷5×4-2×2)

Step 2: Within the Bracket, solve the division section first
4(10+15÷5x4-2×2)

Step 3: Next, within the bracket itself, solve the multiplication
4(10+3×4–2×2)

Step 4:Next, within the bracket, solve the addition
4(10+12-4)

Step 5: At last, within the bracket, solve the subtraction:
4(22-4)

Once the bracket is solved, pick up the number from the outside and solve the ‘Of’ section by multiplication:
=4×18
Answer=72

So,the result of 4(10+15÷5×4-2×2)= 72

You can solve even the most complicated mathematical questions using this BODMAS rule of Brackets of Division, Multiplication, Addition and Subtraction.

Here is the order of operations rule in BODMAS:

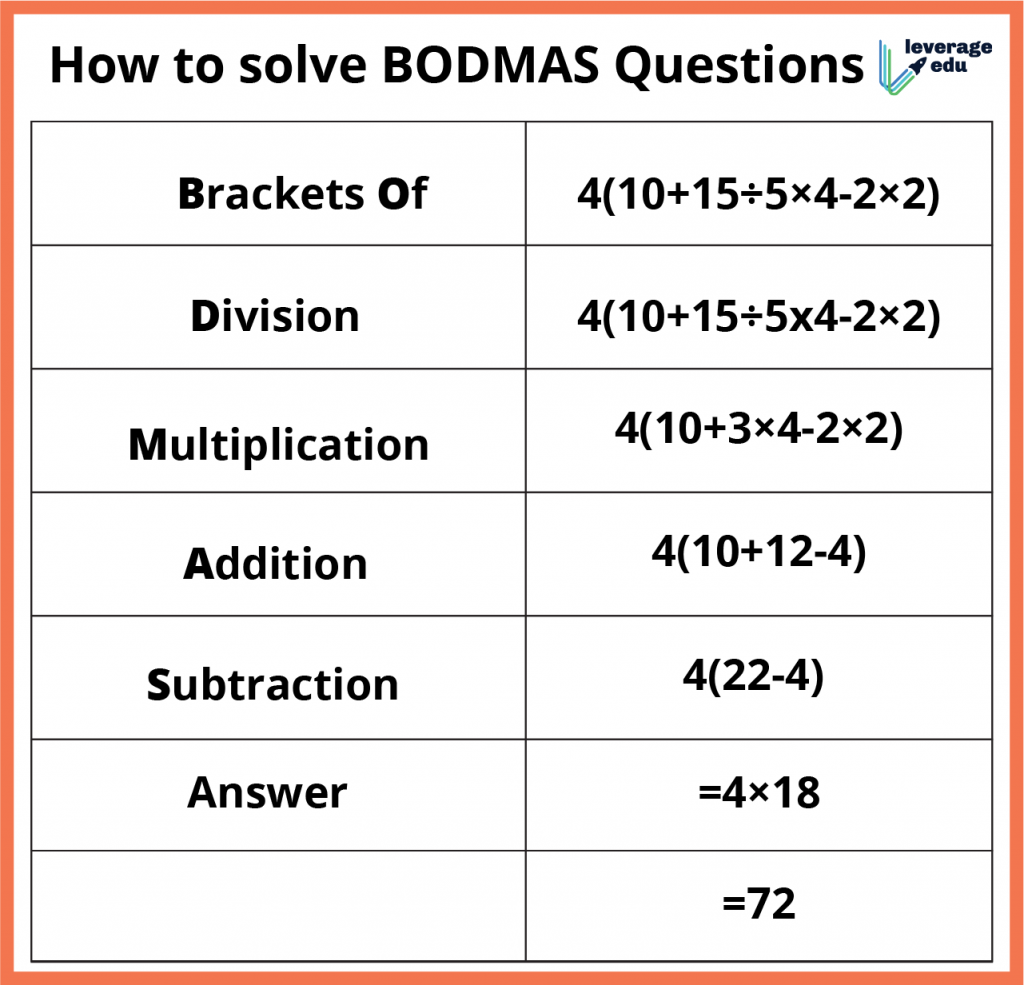
BODMAS Rules

As per the BODMAS rule, the first thing you need to do while solving a question is the simplification of brackets. But do you know how to simplify brackets? Don’t worry, let’s discover ways of removing brackets and expanding an expression with the help of an example:

Suppose we have an equation x (y + z), to open brackets and expand the terms, we will use the Distributive Property which states that a (b + c) = ab + bc. Therefore the given equation will become: x (y + z) =xy + xz.

Common Mistakes to Avoid in BODMAS Questions

Even though BODMAS simplifies solving complex expressions, there are a few common mistakes that people make while applying it:

Mistake 1: Ignoring the Order of Operations

One of the most frequent errors is performing addition or subtraction before multiplication or division. This leads to incorrect results.

Mistake 2: Incorrectly Handling Nested Brackets

When dealing with nested brackets, always begin with the innermost bracket. Skipping this step can disrupt the entire calculation.

Mistake 3: Performing Operations Out of Sequence

People often solve operations in a random order. For example, solving multiplication before division or adding before subtracting.

Real-Life Applications of BODMAS

Understanding BODMAS is not just important for academics. It is also applied in many real-world situations:

• Financial Calculations: When calculating interest, tax, or profit margins, BODMAS helps ensure that the operations are done correctly. For example, calculating compound interest involves exponents, while addition, subtraction, multiplication, and division might be involved in tax calculations.
• Engineering and Construction: Engineers use BODMAS to solve complex equations related to load-bearing calculations, material strength, and structural designs.
• Computing and Algorithms: In programming and data science, BODMAS is essential for writing algorithms that process complex mathematical operations.

BODMAS Examples

We hope the simplification of brackets is clear to you now. Let us look at some complex examples for a better understanding.

Example 1: Expand and simplify 9 (5 + 3)

Solution
In question 9 (5 + 3) let us use the Distributive Property [a (b + c) = ab + bc]
9 (5 + 3) = 9 × 5 + 9 × 3
= 45 + 27
= 72

Therefore, the answer is 72.

Example 2: Expand and simplify 11 (2y+ 3)

Solution:
We will again use the Distributive Property [a (b + c) = ab + bc] to proceed with question 11 (2y + 3). The equation will become:
11 (2y + 3) = 11 × 2y + 11 × 3
= 22y + 33

Therefore, the answer is 22y + 33!

BODMAS Worksheet for Class 10

We have prepared an exclusive worksheet for BODMAS questions for class 10 to practice the BODMAS rules in a better way:

Must Read: Maths Formulas for Class 10

Solved BODMAS Questions and Answers

BODMAS is an important topic of Maths which is a part of class 8 Maths, class 10 Maths and class 9 ICSE Maths. Thus to explain this topic better, we have solved a few BODMAS questions for you, have a look:

Solved BODMAS Question 1

Solved BODMAS Question 2

Find the value of ‘a’ using the concept of BODMAS.
42 ÷ 2 + a × 3 − 22 = 8

Solution:
42 ÷ 2 + a × 3 − 22 = 8
Using BODMAS, we will first work on the division
21 + a × 3 – 22 = 8
Next, we will do the rearrangement of terms, followed by subtraction
a 3 – 22 + 21 = 8
a × 3 – 1 = 8
Taking -1 to the other side
a × 3 = 8 + 1
a × 3 = 9
a = 9/3
a = 3

The value of a is 3!

Solved BODMAS Question 3

Find the value of 3+3 of 3÷3 of 3×3

Solution:
To understand the question better, let us add brackets to this problem
Therefore, 3 + 3 of 3 ÷ 3 of 3 × 3 will become 3 + (3 of 3) ÷ 3 of 3 × 3
Using BODMAS, we will first work on brackets and of
3 + 9 ÷ 3 of 3 × 3
3 + 3 of 3 × 3
3 + (3 of 3) × 3
3 + 9 × 3
As per the BODMAS rule, we will solve multiplication first
3 + 27
= 30

Therefore, the answer is 30!

Solved BODMAS Question 4

In equation 9 + 7 × 1= 5 − 3 which pair of signs should we interchange to make it correct? The available options are + with ×, + with –, × with – or + with –.

Solution:
For the given 4 options, the only × with – is making the equation correct. Let us understand and verify it by replacing these signs in the above-mentioned BODMAS question.

Original Equation: 9 + 7 × 1= 5 − 3
New Equation: 9 + 7 − 1 = 5 × 3

Using the BODMAS rule, we would first work on the multiplication
9 + 7 − 1 = 15
16 − 1 = 15
15 = 15

Hence verified that × with – is the correct choice!

Solved BODMAS Question 5

Solved BODMAS Question 6

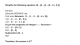
Solved BODMAS Question 7

Find the value of x in the following equation 6162 + x + 3330 = 2545

Solution:
6162 + x + 3330 = 2545
We will first rearrange the equation
6162 + 3330 + x = 2545

Using the BODMAS rule, we will first do the addition
9492 + x = 2545

Shifting 9492 to the other side of the equation
x = 2455 – 9492
= – 6947

Therefore, the answer is – 6947.

Solved BODMAS Question 8

Find the value of 6 ÷ 2 + 7 × 4

Solution:
As per the BODMAS rule, we will start solving the equation by division:
6 ÷ 2 + 7 × 4
3 + 7 x 4

The next operation that falls next as per the BODMAS rule is Multiplication
3 + 7 x 4
3 + 28
= 31

Therefore, the answer is 31.

Solved BODMAS Question 9

Also Read: How are you finding these BODMAS questions? Try out some Algebra Questions as well!

Solved BODMAS Question 10

Simplify [72 – 12 ÷ by 3 – 2 ]+ ( 18 – 6) ÷ 4

Solution:
To simplify the given BODMAS question, we can start by operating the brackets. As brackets further involve division and subtraction, we will division
[72 – 12 ÷ by 3 – 2] + ( 18 – 6) ÷ 4
[72 – 4 – 2] + ( 18 – 6) ÷ 4
66 + 12 ÷ 4

Now we will first work on the division first
66 + 12 ÷ 4
66 + 3
= 69

Therefore, the simplified value is 69!

Find the value of 40 – [20 – {14 – (16 – 6 x 4 – 2)}]

Solution:
As per the BODMAS rule, we will start solving the question by working on the brackets. Within the brackets, we will start with Multiplication followed by subtraction
40 – [20 – {14 – (16 – 6 x 4 – 2)}]
40 – [20 – {14 – (16 – 24 – 2)}]

Property of integers suggests, – and – become +, therefore
40 – [20 – {14 – (16 – 26)}]
40 – [20 – {14 – (-10)}]

Again using – and – become +
40 – [20 – {14 + 10}]
40 – [20 – 24]
40 – [-4]
40 + 4
= 44

Therefore, the answer is 44!

BODMAS Questions For Class 4

Class 4 is an excellent stage to introduce the basics of BODMAS (Brackets, Orders, Division, Multiplication, Addition, Subtraction). Learning BODMAS helps class 4 students to solve math problems systematically and prepares them for more complex calculations in higher classes. Here are questions for Class 4 for BODMAS practice:

• (4+7)*3=
• 5+(18/ 9*2)=
• 12- (2*5)=
• (50/10)-2=
• (30-12)(12-3)=
• (8-6)+(5*5)=

These easy BODMAS questions help Class 4 students build confidence and develop a clear understanding of the order of operations in mathematics.

BODMAS Questions For Class 5

BODMAS in Class 5 to help students learn the correct order of operations while solving any mathematical problems. Understanding BODMAS at this level forms a strong foundation for future mathematical concepts. Here are a few BODMAS questions for class 5 to practice:

• 33-9+40-(30+15) =
• 33-9+40+25-(30+15) =
• 3+21 x 6-(24-4) =
• 3+21 x 6-(24-4) x 2 =
• (62 ÷ 2 – 3) x 3 +6 =
• (62 ÷ 2 – 3) x 3 +6 x 2=
• 0.3+0.8+0.2=
• 30+30×0+1=

BODMAS Questions For Class 6

These BODMAS questions for Class 6 will help improve your understanding and strengthen your ability to apply the rule effectively in exams and real-life scenarios. Here are the BODMAS questions for Class 6 for your practice:

Solve the BODMAS operation:

• 35/7x(7 – 4)+2 =
• 30/5 + 5 (5×6)19+4 =
• 3 – 2 + 3 + 7x(16/8) =
• 5×2 + (11 + 8) – 18/9 =
• (11 – 8)x3 + 7 + 27 – 3 =
• (12/3) + 3 + (16 – 7)x4 =
• 10 – 7 + 9×5 + 28/4 =
• 6/3 + 24 – 25/5 =
• 4 + 2×18/6 – 9 =

BODMAS Questions for Class 7

BODMAS is a crucial concept for Class 7 students, enabling students to tackle complex mathematical expressions systematically. Mastering BODMAS at this level enhances problem-solving skills and builds confidence for advanced topics. Here are five BODMAS questions for class 7:

1. Solve: (8+2)×5−10÷2(8 + 2) \times 5 – 10 \div 2(8+2)×5−10÷2
2. Calculate: 15+[6÷(3×2)]−415 + [6 \div (3 \times 2)] – 415+[6÷(3×2)]−4
3. Find the result: 40÷(5+3)×2+640 \div (5 + 3) \times 2 + 640÷(5+3)×2+6
4. Evaluate: [12×(6+4)]−(20÷4)[12 \times (6 + 4)] – (20 \div 4)[12×(6+4)]−(20÷4)
5. Simplify: 18+(16÷4×2)−1018 + (16 \div 4 \times 2) – 1018+(16÷4×2)−10

BODMAS Questions for Class 8

By Class 8, students are expected to solve more advanced BODMAS problems, integrating higher-order operations and larger numbers. Practicing these questions prepares them for competitive exams and real-world mathematical challenges. Below are five engaging BODMAS questions for Class 8:

1. Simplify: [25÷(5+5)]×8−12[25 \div (5 + 5)] \times 8 – 12[25÷(5+5)]×8−12
2. Calculate: 48−(16÷4)×(3+1)48 – (16 \div 4) \times (3 + 1)48−(16÷4)×(3+1)
3. Solve: (100−45)÷5+(8×3)(100 – 45) \div 5 + (8 \times 3)(100−45)÷5+(8×3)
4. Evaluate: 36+[12×(4−2)]−1836 + [12 \times (4 – 2)] – 1836+[12×(4−2)]−18
5. Find the result: [50÷10+6]×3−5[50 \div 10 + 6] \times 3 – 5[50÷10+6]×3−5

These questions progressively build skills while reinforcing the importance of the BODMAS rule in calculations.

Order Of Operations

Now, Let’s check out the order of operations for solving BODMAS questions:

Why don’t you take a break from these BODMAS questions and solve a few Probability Formulas & Questions for a change?

FAQs

Find Out How Smart Are you With These Trick Questions!

We hope that this blog on BODMAS questions gave you a better understanding of the concept. It is advised to take expert guidance before selecting a stream or course. Leverage Edu is a one-stop solution for all admissions and career-related queries, reach out to us by booking a free E-meeting session!