Compound Interest Questions & Formulas

Are you struggling with the complex Compound Interest questions that often appear in competitive exams? Are you as bewildered as you felt during your school days? Compound Interest is as vital for acing a competitive exam as it was in grabbing a perfect score in mathematics. The fear of solving those lengthy calculations has not yet left us but if you want to qualify for an exam or aspire to get a tantalizing score in math, various tricks can even help you in cracking compound interest. Gaining insights into the topic will not only help you outshine with your marks but also understand the day-to-day financing fundamentals. So, for all the students and candidates preparing for several exams, here is a blog which aims to enlighten you with important Compound Interest questions and their key concepts and formulas. 

Must Read: Trigonometry Formulas

Concept & Formula

Have you wondered why parents are inclined toward a high-interest rate value while choosing a Fixed Deposit or Recurring Deposit? Interest plays an important role on both the part of a lender and borrower. Thus, everyone strives to get a better deal on their side. Before beginning with the Compound Interest questions, let us first understand the concept.

Compound Interest (CI) is the interest calculated on the initial principal and the collective interest of previous periods of a deposit or loan. You can simply apprehend it as ‘Interest on Interest’. In a compound interest investment, you will earn the interest on the initial principal in the first year and then interest on the principal along with the prior year’s interest in the second year. Simple as that!

Here is an example breaking down the formula in simple terms:

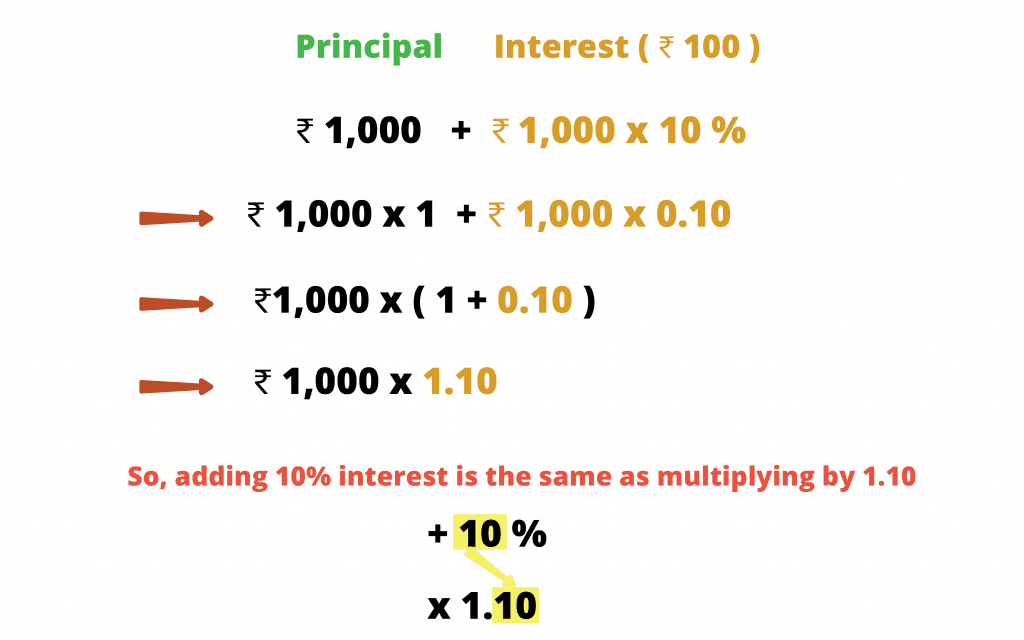
Related Read: Coordinate Geometry for Competitive Exams

Want Free Career Counselling?
Click Here!

Compound Interest Questions 

A mere understanding of the topic will not fetch you the marks you want. Thus, it is important to practice a majority of questions. Regular practice and timely revision can be your success mantra towards achieving your career aspirations. The above-mentioned CI formula gets modified if there is a change in the time period, rate or duration for which CI has to be calculated. Have a look at the table below to understand the variation in the formula as per different factors

Yearly	Factor	r% (per annum)	Time (n years)
Half Yearly	6 months= (6/12)=1/2	Factor x r%= (r/2)%	2n
Quarterly	3 months= (3/12)= 1/4	(1/4) x r%=  (r/4)%	4n
9 months	9 months =(9/12)= 3/4	(3/4) x r%=  (3r/4)%	4n/3
8 months	8 months=  (8/12)= 2/3	(2r/3 x r%=  (2r/3)%	3n/2

Brush up your skills for the quant section, clear all your doubts through our blogs on 

• Mixture and Alligation For Competitive Exams
• Logical Reasoning For Competitive Exams

To test your apprehension of the above-explained concept, below are a few solved and unsolved compound interest questions. 

Q. If the rate is 10% and the principal is 5000, formulate the CI for 2 years if it is compounded half-yearly.

Solution:

If the rate is calculated half-yearly, new rate = 10/2 % = 5% (5%= 1/20)
Given time = 2 years, time for half year= 2×2 = 4 years 
Let’s calculate CI for 4 years at 5 %

5000 x (1/20)= 250
250 x 4= 1000
250 x (1/20)= 12.5 
12.5 x 6= 75
12.5 x (1/20)= 0.625
0.625 x 4= 2.5 
0.625 x (1/20)= 0.03125 
0.03125 x 1= 0.03125 
(1000+75+2.5+0.03125)

= 1077.53125

Q. If the rate is 16(2/3)% =16 and the principal is 216, then calculate the CI for 2 years and 3 years.

Solution:
For 2 years 
16 (2/3)% = 1/6 
216 x 1/6 = 36
36 x 2= 72
36 x 1/6 = 6 
6x 1= 6 
Add both the above values= 72+6 = 78 
CI for 2 years = 78  

For 3 years
216 x (1/6)= 36 
36 x 3 =108
36 x (1/6)= 6 
6 x 3= 18 
6x (1/6)= 1 
1 x 1= 1
Adding the values = (108+ 18+1)= 127 
CI for 3 years = 127

Practice Questions on Compound Interest

• Find the compound interest and the amount on Rs. 8000 at 5%  per annum for 3 years when CI is reckoned yearly?
• Find out the CI on Rs. 5000 at 4% p.a. Compounded half-yearly for 1 ½ year. 
• If Rs. 75,000  are borrowed at CI at the rate of 4% per annum, then, after 2 years the amount to be paid is?
• At the end of three years, what will be the CI at the rate of 10% p.a. on an amount of Rs. 20,000?
• Find the CI on a sum of Rs 1600 for 9 months at 20% per annum, interest is compounded quarterly?
• The CI on a certain sum for 2 years Rs. 41 and the simple interest is Rs. 40 what is the rate per cent?
• A sum of money place at compound interest doubles itself in 4 years. In how many years will it amount to eight times itself?
• Find the least number of complete years in a sum of money put out at 25% compound interest will be more than double of itself?

Thus, we hope that this blog has familiarized you with the basics and formulas as well as some important compound interest questions. If you are struggling with your career choices and do not know which path to choose towards your career, contact us at Leverage Edu and our experts will guide you in mapping your interests as well as skills and finding a suitable field that aligns with your aspirations and preferences.