Circles Class 9 Study Notes

Mathematics is fun to learn and an important subject in a student’s career. Maths is the basis of almost all the things we see or perceive around us, topics like circles; probability and  geometry play a crucial role not only in schools but also in real-life scenarios. In everyday life, we come across a variety of objects which are spherical ( a circle in three dimensions is a sphere) or circular like coins, clocks, wheels of most vehicles, planets and many more. If you are a class 9th student looking to strengthen your skills in circles class 9, then continue reading this post.

Basic Elements of a Circle 

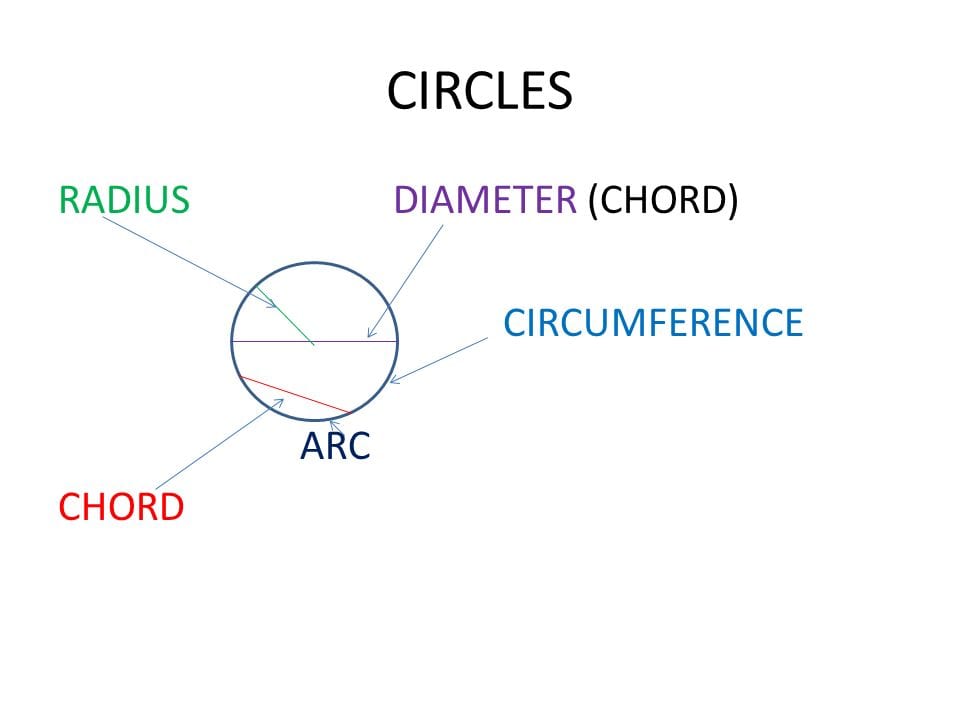
• A circle is basically a combination of all the points in a plane, which are at a determined length from a fixed point in a plane (center of the circle). 
• The line which joins the center and any point on the circle is called the radius of that circle.
• If you take two points say A and B on a circle, then the line segment AB is called a chord of the circle. The chord, which crosses through the center, is called a diameter and is 2 times the radius (diameter = 2 * radius).
• The length of the entire circle is described as its circumference.
• An arc is the connected region of the circumference of the circle. Imagine a circle with center O, now if we have two points namely B and C then the connected region BC is an arc of the circle.
• A chord that divides the circle into two regions is called the segments of the circle. Segments are of two types:
  Minor Segment – It is the region defined by the chord and the minor arc intercepted by the chord.
  Major Segment – It is the region defined by the chord and the major arc intercepted by the chord.

Note: If the lengths of two arcs are equal, then it divides the circle into two semicircles.

Angle Subtended by a Chord at a Point – Circles Class 9

Now to get a better idea about this, imagine a circle with center O and a triangle inside it, while all the edges of the triangle lie on the circle. Now we will see how the size of the chord is associated with the angle defined by it at the center. 

The longer the length of the chord the greater will be the angle subtended by it at O (the center). Likewise, if the length of the chords is the same then the angle subtended by them at the center will be equal and the vice versa is also true.

Have a look at the question below.

The image shown below depicts the above scenario.

Now let us see how we can solve the problem.

Also Read: Class 9 Maths- Heron’s Formula

Circle through Three Points

As we all know, in order to draw a line two points are required and only a single line can pass through any given two points. So now let us see how many points are required to determine a circle.

Consider a straight line XY, then the line that passes through the midpoint of the segment XY and is at 90 degrees to it. Let’s call this line l (X, Y).

Given 3 non-collinear points P, Q, and R the lines l(P, Q), l(Q,R), and l(P,R) are not parallel, because the lines PQ, PR are not parallel, and therefore meet at exactly one point. This point is equidistant from P, Q, R, and is hence the only such point. It is the center of the single circle that passes through these three points.

Thus, we can gather that three points are required to determine a circle. 

[optin-monster-shortcode id=”xf2mlnjiouddzrshykdb”]

Look at the example question below,

Now, for solving this,

Also Read: Class 9 ICSE Maths Syllabus

Angle Subtended by an Arc of a Circle

Now let us assume we have a circle with its center O if you draw two equal chords then what will be the size of their arcs? Well, the simple answer is that they will be equal in length. You can verify or prove this yourself by drawing two chords in a circle (SV and PM) and when one arc is put on the other, one superimposes the other perfectly. Therefore, it can be concluded that two chords of the same length will cut equal arcs and the reverse also holds true.

We hope this blog helped you in understanding the basics of concepts of circles class 9. If you want to learn more or want an insight into different subjects like Chemistry, Biology, English, Physics class 9 then refer to our comprehensive study notes. For any career-related advice reach out to Leverage Edu experts and take a step forward in your journey towards excellence.