3D Geometry Class 11 Study Notes

Class 11 Maths has an introductory chapter to advanced mathematics, chapter 12 Introduction to Three-dimensional Geometry. One of the bases for advanced mathematics, it is a chapter that is not just important for Class 11, but also if you plan to study maths or physics in any form in higher studies. This chapter is also an important part of the syllabus of prominent competitive exams like JEE Advance, Mains, etc. Thus, it is essential for you to understand this chapter in detail, so that you not only score good marks in it but create a solid base for class 12 mathematics concepts. For all such students, here is a blog which aims to guide them about 3D geometry class 11. 

Check full Class 11 Maths Syllabus here!

Basics and Foundations for Three-dimensional Geometry

Some of the important basic topics of this chapter are the cartesian plane, coordinates of a point, ordered pair, one-dimensional coordinate system, and two-dimensional coordinate system. Only when the students understand these bases, then, they will be able to cover for the three-dimensional geometry. 

3D Geometry Class 11: Definitions

Mentioned below are the definitions of important terminologies chapter on 3D geometry class 11- 

Cartesian Plane

Two perpendicular number lines form a cartesian plane. The name comes from Rene Descartes, the French mathematician who made its formal use in mathematics. A point in a plane can be described using the ordered pair of numbers on this plane.

• Using the above plane, we can find the exact location of any point on the plane, also known as the coordinates of a point.
• Ordered pair refers to the exact way in which coordinates of a point are mentioned. It is always x-point followed by y-point in an XY plane. 
• One-dimensional coordinates are simply a number line and extend only in one axis. The location of any point is determined by a single point, stating how far it is from zero (e.g., -10, 7).
• The two-dimensional coordinate system is the same as the cartesian plane, and the coordinates of a point are determined by an ordered pair, as noted above.

Coordinate Axes and Planes

When we talk about three dimensions, there are three different axes instead of two, as in a Cartesian Plane. The Cartesian coordinate system is a rectangular system with three mutually perpendicular lines forming the coordinate axes, the axes being x-axis, y-axis, and z-axis. The resulting three planes as a result of these three axes are called coordinate planes. The planes are XY plane, YZ plane, and ZX plane. The coordinates of a point on a three-dimensional space determined by the three axes are written as an ordered pair (or ordered triplet) (x,y,z). The coordinates of a point in various positions can be, thus:

• Points on the X-axis are (x,0,0)
• Points on the Y-axis are (y,0,0)
• Points on the Z-axis are (z,0,0)
• Points on the XY-plane are (x,y,0)
• Points on the YZ-plane are (0,y,z)
• Points on the XZ-plane are (x,0,z)
• Points anywhere in the 3-d space are (x,y,z).

Octants

Octants are the eight spaces in which the three coordinate planes divide the space. These are –

1. Octant I: x,y,z are all positive.
2. Octant II: x is negative; y and z are positive.
3. Octant III: x and y are native, z is positive.
4. Octant IV: x and z are positive, y is negative.
5. Octant V: x and y are positive, z is negative.
6. Octant VI: y is positive, x and z are negative.
7. Octant VII: x,y,z, are all negative.
8. Octant VIII: x is positive, y and z are negative.

Distance Formula

It is essential to remember various distance formulas because they will be repeated across the chapter on 3D geometry class 11. Some of them are as follows:

PQ, the distance between points P (x1, y1, z1) and Q (x2, y2, z2) is as follows: 

The distance of any point P (x,y,z) from the origin (0,0,0) is:

OP =

Practice Question:
What is the distance between two given points P(2,3,4) and Q (6,4,3)
Answer:
PQ = √(x₂–x₁)² + (y₂-y₁)² + (z₂-z₁)²
=√(6-2)²+(4-3)²+(3-4)²
=√16+1-1
=√16
=4

Section Formula

Section Formula happens to be among the top-most important concepts of this chapter. The line segment joining P (x1,y1,z1) and Q (x2,y2,z2) is divided internally or externally by point R in the ratio of m:n. The coordinates of the point R will be 

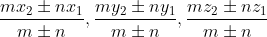
Mid-point coordinates for line segment that joins P (x1,y1,z1) and Q (x2,y2,z2) are:

The centroid of the triangle with vertices (x1,y1,z1), (x2,y2,z2) and (x3,y3,z3) has the following coordinates:

Practice Question: Find the coordinates of a point that divides a line segment into two equal halves?

Answer: Let PQ be a line segment where P (x1, y1, z1) and Q (x2, y2, z2) are the endpoints. Let C be the mid-point of the line segment AB. Here, m = n = 1. Placing values in the formula, C (x, y); x = (x1 + x2) ⁄ 2, y = (y1 + y2) ⁄ 2.

Practice Question: Find the coordinates of the points A and B that divide the line segment PQ {P(2,4,1) and Q(1,3,2)} internally and externally both in the ratio 3:5.

Solution: The coordinates of A (dividing PQ internally), x = (3×1 + 5×2) ⁄ (3 + 5) = (3 + 10) ⁄ 8 = 13⁄8, y = (3×3 + 5×4) ⁄ (3 + 5) = (9 + 20) ⁄ 8 = 29⁄8, z = (3×2 + 5×1) ⁄ (3 + 5) = (6 + 5) ⁄ 8 = 11⁄8. The coordinates are (13⁄8, 29⁄8, 11⁄8).

Coordinates of A (dividing PQ externally), x = (3×1 − 5×2) ⁄ (3 − 5) = (3 − 10) ⁄ −2 = 7⁄2, y = (3×3 − 5×4) ⁄ (3 − 5) = (9 − 20) ⁄ −2 = 11⁄2, z = (3×2 − 5×1) ⁄ (3 − 5) = (6 − 5) ⁄ −2 = −1⁄2. The coordinates are (7⁄2, 11⁄2, −1⁄2).

Direction Ratios

The chapter on 3D Geometry class 11 defines the direction ratios as simply the numbers that are proportional to the direction cosines. Consider p,q,r to be direction cosines and m,n,o to be direction ratios of a line. Here,

Consider a1,b1,c1, and a2,b2,c2 as direction cosines of two lines. Consider p to be the acute angle between them. Then:

cosp = |a1a2 + b1b2+ c1c2 |.

Consider a,b,c to be direction cosines of a line. Then:

Getting a hold of this simple formula will help you in creating a strong grip over many concepts of mathematics that are essential syllabus of mathematics of class 11 and 12.

Cartesian Equation where a plane passes through the intersection of planes P1x + Q1y + R1z + S1 = 0 and P2x + Q2y + R2z + S2 = 0 is,

(P1x + Q1y + R1z + S1) + l(P2x + Q2y + R2z + S2) = 0

Practice Question: A line makes equal angles with all of the coordinate axes. Find it’s direction cosines
Answer: The angles that it makes be denoted as α, β, γ with the coordinate axes. The direction cosines will therefore be cos α, cos β, cos γ. We know that l = cos α, m = cos β, n = cos γ
Therefore, we use the relation l2 + m2 + n2 = 1
So, (cos α)2 + (cos β)2 ­­+ (cos γ)2 = 1
because we are provided with the condition that it makes equal angles, cos α = cos β = cos γ
Thus, 3(cos α)2 = 1
(cos α)2 = 1/3
cos α= (1/3)1/2 
Hence, all the direction cosines are given by (1/3)½

Important Questions

• Determine the vector equation of a line X that is passing through coordinates (6,7,10) and (-2, 1, 4) 
• Find the angle that lies between the planes 12x + 12y – 12z = 8 and 13x- 26y + 12z= 17 
• Determine the cosines of a line Y if it is making angles 90°, 45° and 135° with the x,y and z axes. 
• If the given coordinates of the vertices of a triangle are (4, 5, 7), (-2, 1, 4) and (-6,-6,-3). Find the direction cosines of the sides of the given triangle. 
• Determine the vector as well as the cartesian equation of the lines passing through centre and points (6, 2, 5) 
• Find out the angles formed between the Planes 12x + 2y -12z = 15 and 23x – 25y- 12z= 27 

Thus, we hope that through these study notes of chapter on 3D Geometry class 11, we have assisted you in understanding this chapter. Pursuing the right course with respect to long term career goals, helps one kick start their career in the right direction. Our experts at Leverage Edu are here to guide you in selecting the right course by analysing your interest and choices. Hurry up! Book an e-meeting with us today.