Mechanical Properties of Solids

In all engineering construction, the fundamental components of a machine or structure are designed by keeping in mind the mechanical properties of solids. This is so that the parts are proportioned to resist the forces imposed on them. Be it the floors of a building, walls of a pressure cooker, or wings of an aircraft, the right choice of materials and dimensions can make or break the parts! A sound understanding of these properties and the chapter mechanical properties of solids will help you grasp the concepts of subsequent chapters with ease and strengthen your grip over class 11 physics. So, let’s begin to learn this chapter from the class 11th Physics NCERT solutions! 

[powerkit_toc title=”This Blog Includes:”]

Intermolecular Forces, Elasticity, and Plasticity 

Intermolecular Forces – As we know, solids are made up of atoms and molecules packed close to each other. The arrangement is such that each molecule exerts a force on its neighbouring molecule. These forces are known as intermolecular forces. 

Elasticity: It is the ability of a deformed body to return to its original dimensions (shape, size, and volume) when the external forces are removed. Bodies that show elasticity are called elastic bodies. Examples of perfectly elastic bodies: Quartz Fiber, Phosphor Bronze 

Plasticity: It is the ability of a body to undergo permanent deformation (in shape, size, or volume) when the deforming forces are removed. Bodies that show attributes of plasticity are called plastic bodies. Examples of plastic bodies: Wax, Clay, Plasticine 

Class 11 Physics Chapter 7 – System of Particles and Rotational Motion

Stress, Strain, and Hooke’s Law

Stress 

In the mechanical properties of solids, stress is defined as the restoring force per unit area. Hence, Stress in SI units is N/m² or Pascal (Pa). Types of Stresses in Solids: 

• Normal Stress/ Longitudinal Stress- It is defined as the type of stress that occurs when an axial force is applied perpendicular to the surface. Eg. Pressure. Normal stresses can be further divided into: 

Tensile Stress	Compressive Stress
The restoring force per unit area is when a body is stretched by two equal and opposite forces acting perpendicularly to the cross-sectional area.	The restoring force per unit area is when the body is compressed by opposing forces.

• Shearing Stress/ Tangential Stress– It is the type of stress that occurs when the restoring force acts parallel or tangentially to the surface of the body. Eg. Cutting a piece of paper with scissors. 

Class 11 Physics Chapter 3 – Motion in a Straight Line

Strain 

Strain is defined as the measure of deformation or elongation of an object when acted upon by an external force. Since it deals with the relative change in length or shape of an object, it is a dimensionless quantity. Types of strains are: 

• Longitudinal Strain/Tensile Strain- As the name suggests, longitudinal strain occurs when the deforming forces produce a change in the body’s length
• Shearing Strain: The strain produced when tangential stress causes an angle tilt in the body
• Volumetric strain- The strain produced when the deforming forces cause only a change in the volume of a body

Hooke’s Law 

As per the chapter, Mechanical Properties of Solid, Hooke’s law expresses the relationship between the two main mechanical properties of solids- stress, and strain. It states that, within an elastic range, the strain within an object (consider a spring in this case) is proportionate to the stress applied to it. Therefore, the ratio of stress to strain is given by a constant. This constant is called the modulus of elasticity. Hence, As strain is just a number, SI units of the modulus of elasticity are the same as stress (N/m² or Pascal (Pa)). 

Bulk Modulus

The ratio of hydraulic stress to the corresponding hydraulic strain is called bulk modulus. It is denoted by a symbol 

B. B = – p/(ΔV/V)  

The negative sign indicates the fact that with an increase in pressure, a decrease in volume occurs. That is, if p is positive, ΔV is negative. Thus for a system in equilibrium, the value of bulk modulus B is always positive. SI unit of bulk modulus is the same as that of pressure i.e., N m–2 or Pa.

Mechanical Properties of Solids: Stress-Strain Curve

As given in the chapter mechanical properties of solids, a stress-strain curve is a reliable way to determine and evaluate the mechanical properties of solids. It can help you understand the load-bearing capacity or tensile strength of a material. Let’s take metals for this example. 

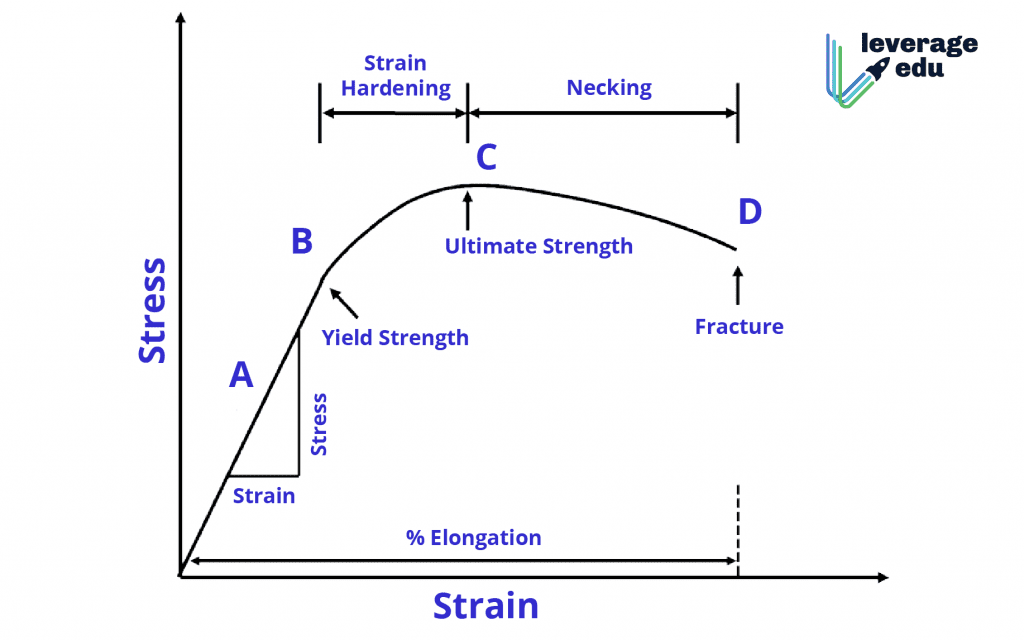
● A– Shown as a linear curve. This is the region where the material obeys Hooke’s law, i.e. The material regains its original position when the load is removed

● B– Also known as the elastic limit or yield point. The stress at this point is called the yield strength of the material

● B to C– This curve denotes the region of plastic deformation 

● C– Point C shows the ultimate strength or tensile strength of the material 

● D– Any additional strain beyond this point may lead to permanent fracture of the material

Modern Physics

Important Ratios Defining Mechanical Properties of Solids

Young’s Modulus– Within the elastic limits of a thin wire or rod, it is defined as the ratio of longitudinal stress to longitudinal strain. 

Bulk Modulus- Measures the ability of a substance to withstand compression from all sides. It is defined as the ratio of normal stress to volumetric strain 

Shear Modulus/ Modulus of Rigidity- According to the mechanical properties of solids chapter, it is defined as the ratio of shear stress to shear strain

Poisson’s Ratio- It is defined as the ratio between lateral strain (change in diameter) and longitudinal strain (change in length)

Basic Physics Formulas & Notes for Competitive Exams

Mechanical Properties Of Solids Summary 

1. Stress is the restoring force per unit area and strain is the fractional change in dimension. In general there are three types of stresses (a) tensile stress — longitudinal stress (associated with stretching) or compressive stress (associated with compression), (b) shearing stress, and (c) hydraulic stress. 
2. For small deformations, stress is directly proportional to the strain for many materials. This is known as Hooke’s law. The constant of proportionality is called modulus of elasticity. Three elastic moduli viz., Young’s modulus, shear modulus and bulk modulus are used to describe the elastic behaviour of objects as they respond to deforming forces that act on them. A class of solids called elastomers does not obey Hooke’s law.
3. When an object is under tension or compression, the Hooke’s law takes the form 
   F/A = YΔL/L 
   where ΔL/L is the tensile or compressive strain of the object, F is the magnitude of the applied force causing the strain, A is the cross-sectional area over which F is applied (perpendicular to A) and Y is the Young’s modulus for the object. The stress is F/A. 
4. A pair of forces when applied parallel to the upper and lower faces, the solid deforms so that the upper face moves sideways with respect to the lower. The horizontal displacement ΔL of the upper face is perpendicular to the vertical height L. This type of deformation is called shear and the corresponding stress is the shearing stress. This type of stress is possible only in solids. In this kind of deformation the Hooke’s law takes the form
   F/A = G × ΔL/L 
   where ΔL is the displacement of one end of object in the direction of the applied force F, and G is the shear modulus.
5. When an object undergoes hydraulic compression due to a stress exerted by a surrounding fluid, the Hooke’s law takes the form 
   p = B (ΔV/V), 
   where p is the pressure (hydraulic stress) on the object due to the fluid, ΔV/V (the volume strain) is the absolute fractional change in the object’s volume due to that pressure and B is the bulk modulus of the object.

Thus, we hope that through this blog about the mechanical properties of solids, you are now well-versed with the chapter. If you want to explore trending career options in which you can kick start your career, reach out to our experts at Leverage Edu and they will assist you the best. Book an e-meeting.