What is the Difference Between Variance and Deviation?

The difference between variance and deviation is that the deviation (aka standard deviation) indicates how far individual points are from the average (mean). It’s expressed in squared units (e.g., metres, years). Whereas variance represents the average squared distance from the mean. It’s expressed in squared units (e.g., meters², years²).

What is Variance?

Imagine a collection of leaves. Variance tells you how far each leaf is from the average leaf size. High variance means leaves vary greatly in size, while low variance indicates similar-sized leaves. In statistics, it’s the average of squared distances from the mean (average value). This table provides a comprehensive overview of variance, covering its definition, formula, properties, notation, interpretation, and calculation methods.

Definition	Variance is the expected value of the squared deviation of a random variable from its mean.
Formula	Var(X) = E[(X – E(X))^2]
	Var(X) = E[X^2] – (E[X])^2
Properties	Additive property: Var(X + C) = Var(X), where C is a constant. Constant multiple property: Var(CX) = C^2.Var(X), where C is a constant. Linear transformation property: Var(aX + b) = a^2.Var(X), where a and b are constants. Sum of independent variables property: Var(X1 + X2 + … + Xn) = Var(X1) + Var(X2) + … + Var(Xn), if X1, X2, …, Xn are n independent random variables.
Notation	Variance is symbolically represented by σ^2, s^2, or Var(X).
Interpretation	A higher variance indicates a greater spread of data points from the mean, suggesting higher variability or dispersion. Lower variance implies data points are closer to the mean, indicating less variability or dispersion.
Relationship with standard deviation	Variance is the square of the standard deviation, i.e., variance = (standard deviation)^2 = σ^2.
Calculation	1. Compute the mean of the data set. 2. Subtract the mean from each data point to get deviations. 3. Square each deviation. 4. Take the average of the squared deviations to get the variance.

Must Read:Difference Between Parallel and Perpendicular

What is Deviation?

The standard deviation measures the spread of data from the mean, reflecting typical deviations. It returns to its original units, making it popular for variability assessment. Closed data to the mean yields a low deviation; spread data indicates a high variance. It’s affected by all values, making it independent of origin but not scale. It’s crucial in advanced statistical analyses. This table summarises the key aspects of standard deviation, including its definition, calculation formula, properties, interpretation, application, and an example scenario.

Definition	Standard Deviation measures the extent of variation or dispersion from the mean in a dataset. It indicates the typical deviation from the mean.
Calculation Formula	Standard Deviation (σ) = √[Σ(x – μ)^2 / N] Where: σ is the standard deviation- x is each data point- μ is the mean of the dataset- N is the total number of data points
Properties	The standard deviation is independent of the origin but not of the scale.
	It is the square root of the variance, the average of the squared differences from the mean.
Interpretation	Let’s consider calculating the standard deviation for the number of gold coins on a pirate ship. The population standard deviation formula is used if the population size (total pirates) is known. If only a sample is available, the sample standard deviation formula is applied.
Example	Let’s consider calculating the standard deviation for the number of gold coins on a pirate ship. The population standard deviation formula is used if the population size (total pirates) is known. If only a sample is available, the sample standard deviation formula is applied.

Must Read :Difference Between Marginal Cost and Average Cost

What is the Difference between Variance and Deviation

This table provides a concise overview of the differences between Variance and Standard Deviation, covering aspects such as definition, calculation formula, expression, mathematical notation, indicator of, measure of, nature of measure, calculation method, and application.

Variance vs Deviation	Variance	Standard Deviation
Definition	Numerical value describing the variability of observations.	Observations’ dispersion within a dataset.
Calculation Formula	Average of squared deviations.	Square root of the mean square deviation.
Expression	Expressed in squared units.	Expressed in the same units as the data.
Indicator of	Perfect indicator of individual spread within a group.	Perfect indicator of observation spread in a dataset.
Measure of	Dispersion of values relative to their mean.	How far numbers are spread from their average in a dataset.
Nature of Measure	Measures absolute variability.	Determines the size of data spread.
Calculation Method	Calculated by taking the square root of the variance.	Calculated by taking the average of the squared deviations of each value from the mean.

Similarities Between Variance and Deviation

Variance and deviation are both concepts used in statistics to describe how spread out data is from its average value (the mean).Imagine a target with the bullseye being the mean. Deviation tells you how far each dart lands from the bullseye, while variance tells you the average squared distance of all the darts from the bullseye. Here are the most important similarities between them:

• Both variance and deviation quantify how far individual data points fall from the mean. A higher value indicates a larger spread, meaning data points are further from the average.
• They are often used together to understand the distribution of data. Variance provides the squared distance on average, while deviation gives an idea of the raw distance from the mean.

Variance and Deviation Formulas 

The average square distance between each data value and the mean value represents the data set’s variance, The distribution of data values around the mean is defined by the standard deviation. The population, as well as the sample variance formula, is given by:

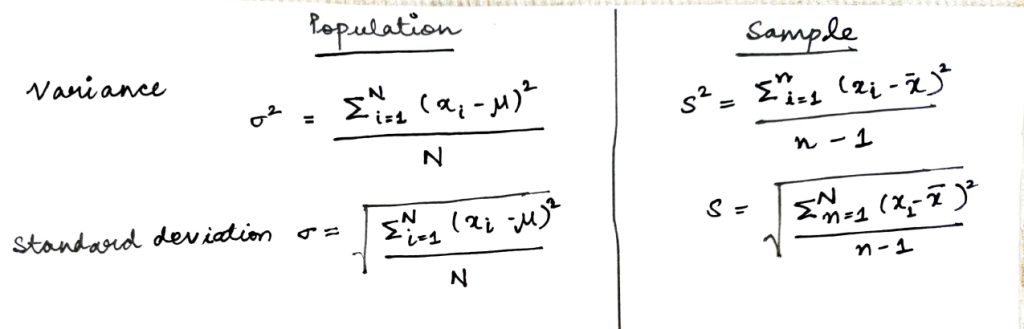
Here,

• σ2 = Population variance
• N = Number of observations in population
• Xi = ith observation in the population
• μ = Population mean
• s2 = Sample variance
• n = Number of observations in the sample
• xi = ith observation in the sample
• σ = Population standard deviation
• s = Sample standard deviation

Application of Variance and Deviation in Real Life

Variance and deviation, though similar, serve distinct purposes in real life.Below we have mentioned some real life applications of variance and standard deviation and explained them using examples:

• Variance: Imagine a bakery. Ovens might have slight temperature differences. Variance tells you how much bread baking times differ from the average. A high variance means some buns burn while others stay raw.
  
• Standard Deviation (deviation): It’s like a “spread meter.” In the bakery example, the standard deviation tells you how many buns fall within a specific time range around the average baking time. A low deviation indicates consistent baking, while a high one suggests uneven temperatures.

RELATED BLOGS

Blood Relation Reasoning Questions	Questions of Verification of Truth Reasoning
Classification Reasoning Questions	Questions of Arithmetic Reasoning
Questions of Syllogism Reasoning	Questions of Direction Sense Test Reasoning

FAQ’s

This was all about the “Difference Between Variance and Deviation”.  For more such informative blogs, check out our Study Material Section, you can learn more about us by visiting our  Indian exams page.