One app for all your study abroad needs
Factors are numbers that can divide a given number without leaving any remainder. These factors have an important part in algebra, number theory, and problem-solving. Furthermore, they are key in finding common denominators, simplifying fractions, and determining multiples. Read on to learn more about the Factors of 20, the Factor Tree of 20, the Prime Factors of 20, Factor Pairs and Factors of 20 by Division Method.
Table of Contents
What are the Factors of 20?
The factors of 20 are all the integers that divide evenly into 20. Moreover, here is the complete list of factors of 20:
1, 2, 4, 5, 10, 20
As you can see, each of these factors is divided into 20 with no remainder:
- 20 / 1 = 20
- 20 / 2 = 10
- 20 / 4 = 5
- 20 / 5 = 4
- 20 / 10 = 2
- 20 / 20 = 1
However, it is important to remember that 1 and the number itself are always considered factors of any number. Hence, 1 and 20 are automatically included in the Factors of 20.
Also Read: Lateral Area of Cylinder: Formula, Example and More!
What is the Factor Tree for 20?
A factor tree is a visual representation of how a number can be broken down into its Prime Factors. Therefore, to create a Factor tree for 20, you will need to continuously divide it by prime numbers until we reach 1. Prime numbers are numbers greater than 1 that have only two factors: 1 and itself.
Here’s how the factor tree for 20 looks:
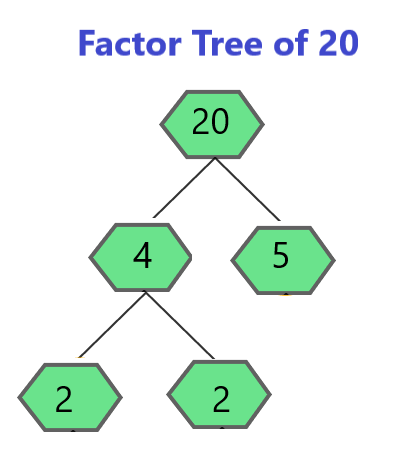
You need to start by dividing 20 by 2 which is a Prime number thus resulting in 10. Since 10 is not a Prime number, we can further divide it by 2. Therefore, this process continues until we reach Prime factors which are 2 and 5.
Also Read: What is the Difference Between Volume and Area?
Factors of 20 By Prime Factorization Method
Prime factors are the building blocks of any composite number (a number with more than two factors). As we saw in the factor tree, the prime factors of 20 are 2 and 5.
Hence, the Prime factorization of 20 is 2 x 2 x 5 or 2² x 5. Hence, this means that 20 is the product of two twos and one five.
Also Read: What is the Difference Between Parallelogram and Rectangle?
Factors of 20 by Division Method
Furthermore, another method to find the Factors of 20 is via the Division Method. This is the step-by-step procedure:
- Start by dividing 20 by the smallest prime number which is 2. If the division is even with no remainder, then 2 is a factor of 20. In this case, 20 divided by 2 is 10.
- Since 10 is not a Prime number you need to continue dividing it by 2. 10 divided by 2 is 5.
- We reach a Prime number, 5. Hence, 5 is a Factor of 20.
Also Read: Curved Surface Area of Cylinder: Formula, Examples
- Now, check if 5 divides 20. Since 20 divided by 5 is 4, 5 is another factor.
- We are left with 4. Since 4 divided by 2 is 2, both 4 and 2 are factors of 20.
- Finally, we reach 1. Dividing by 1 does not result in a new factor, but it confirms that 1 is always a factor of any number.
Therefore, by following these steps, we arrive at the same set of factors as before which are 1, 2, 4, 5, 10, and 20.
Also Read: Cross-Sectional Area of Different Shapes with Formula
What are Factor Pairs for 20?
Factor pairs of a number are all the unique combinations of two numbers that multiply to give that specific number.
In the case of 20, here are all the Factor pairs:
- 1 x 20
- 2 x 10
- 4 x 5
- 5 x 4
- 10 x 2
- 20 x 1
As you can see, each pair of numbers multiplies to give 20. Moreover, finding Factor pairs can be helpful in different situations, such as finding the area of a rectangle with specific side lengths or understanding divisibility rules.
Related Blogs
I hope this helps! Did you like learning about the Factors of 20? Keep reading our blogs to learn more about the Basic Concepts of Maths!