One app for all your study abroad needs
The Factors of 18 represent the divisibility of the number 18 with a number lower than 18 without leaving any remainder. Furthermore, these factors come into use when solving equations in mathematics and other day-to-day activities such as handling money. Additionally, in this blog, you will learn the Factors of 18, their factor tree, factor pairs and the prime factorisation method.
What are the Factors of 18?
Table of Contents
Factors of a number are integers that divide evenly into that number and leave no remainder. When it comes to 18, these factors include:
- 1
- 2
- 3
- 6
- 9
- 18
Therefore, each of these numbers is divided into 18 entirely.
For instance, 2 goes into 18 nine times (18/2 = 9), and 3 goes into 18 six times (18/3 = 6).
Moreover, it is important to remember that 1 and the number itself are always considered factors of any number.
Also Read: Algebraic Identities: Examples and Chart
What is the Factor Tree of 18?
A factor tree is a visual representation of how a number can be broken down into its prime factors (factors that cannot be further divided by whole numbers).
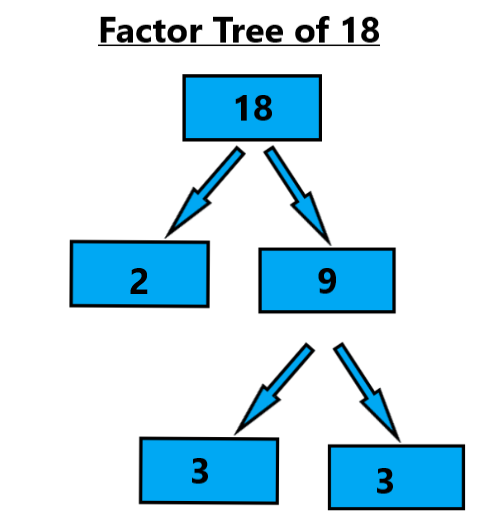
Additionally, here is how you create a factor tree for 18:
- Start with 18 at the top.
- Find the smallest prime number that divides 18. In this case, it is 2.
- Draw a line connecting 18 to 2.
- Divide 18 by 2 (18/2 = 9).
- Since 9 is not a prime number, see if it can be further divided. In this case, 3 divides 9 perfectly (9/3 = 3).
- Draw lines connecting 9 and 3, and 2 and 3.
Furthermore, this process stops here because 3 is a Prime number. The completed Factor tree shows that 18 can be represented as the product of Prime numbers: 2 x 3 x 3.
Also Read: 10 Properties of Determinants: Formulas and Examples
What are Factor Pairs for 18?
Factor pairs are two factors of a number multiplied together to give the original number. Looking at the factors of 18 which are 1, 2, 3, 6, 9, and 18, you can identify three factor pairs:
- 1 x 18
- 2 x 9
- 3 x 6
Thus, each pair, when multiplied results in 18 (1 x 18 = 18, 2 x 9 = 18, 3 x 6 = 18).
For example, if you have 18 cupcakes and want to share them equally among friends, you can consider different group sizes based on the factor pairs. You could share them with 1 friend (1 x 18), split them between 2 friends with 9 cupcakes each (2 x 9), or create groups of 3 friends with 6 cupcakes each (3 x 6).
Also Read: All You Need to Know About HCF and LCM
Factors of 18 By Prime Factorization Method
Prime factorization is a systematic approach to breaking down a composite number (a number with more than two factors) into its Prime factors.
Here is how to find the Factors of 18 using Prime factorization:
- Continuously divide the number by the smallest prime number that divides it evenly. In this case, start with 2.
- Now you see 2 divides 18 (18/2 = 9).
- However, 9 is not a prime number. So, you continue dividing by the next prime number, which is 3.
- Then you see that 3 divides 9 perfectly (9/3 = 3).
Since you have reached a Prime number (3), the process stops. This reveals that the Prime factorization of 18 is 2 x 3 x 3. Therefore, by multiplying these Prime factors together (2 x 3 x 3), you get back to the original number 18.
Related Blogs
I hope this helps! Did you like learning about the Factors of 18? Keep reading our blogs to learn more about the Basic Concepts of Maths!