One app for all your study abroad needs
In geometry, we learn about different dimensional shapes: One-dimensional, two-dimensional and three-dimensional shapes. Three-dimensional shapes have three measurements: length, breadth, and height. The volume of an object refers to the space occupied by three-dimensional objects. In this section, we will cover the volume of a hemisphere with some solved examples.
Table of Contents
What is a Hemisphere?
A sphere is a three-dimensional shape with each point on its surface equidistant from the centre. The term ‘Hemi’ means half as it is denoted by the half of the sphere. A hemisphere is formed when a plane divides a sphere into two equal parts from its centre. So a hemisphere is half of a sphere. Basically, a hemisphere is a three-dimensional object that is flat from one side and has a bowl-like shape on the other side. For example, the earth is a single big sphere divided into two hemispheres: northern and southern.
What are the properties of the Hemisphere?
The hemispheres have the following properties:
- A hemisphere has no edges and vertices.
- A hemisphere has one curved surface and one flat surface.
- A line segment that connects two opposite points on the circumference of the circle and passes through its centre is called the diameter of the hemisphere.
- A line segment from the centre to any of the points on the circumference of the circle is the radius of the hemisphere.
What is the Volume of the Hemisphere?
The volume of a hemisphere refers to the space occupied by a hemispherical object. Any object with a large volume takes up a large space. The volume of a hemisphere can also be described as half the volume of a sphere. The formula is calculated by Archimedes.
Also Read: Difference Between Volume and Area
Formula of Volume of Hemisphere
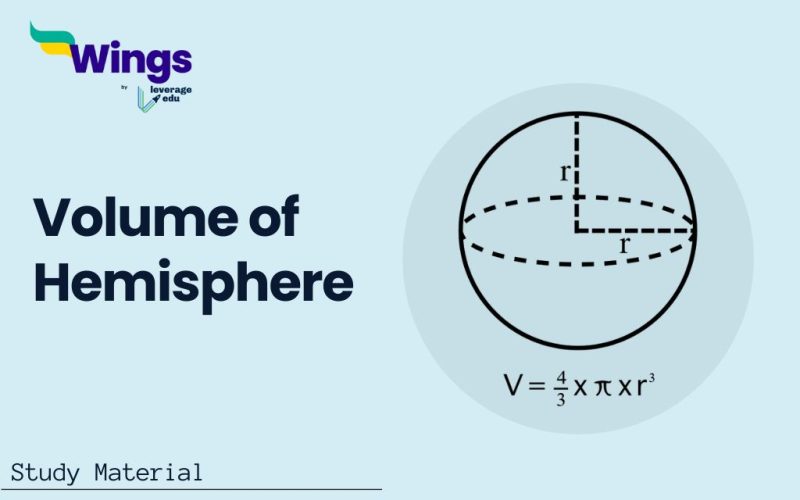
The formula for the volume of a hemisphere is 2πr3/3 cubic units.
Where, π = 3.14
r= radius of the hemisphere
Now, let’s look at how the formula is derived.
The volume of a sphere= 4πr3/3
As the hemisphere is the half of the sphere, the volume will be;
= 1/2 x 4πr3/3
=2πr3/3
How to Find the Volume of a Hemisphere
The volume of the hemisphere can be calculated by using the formula, which is 2πr3/3. So, let’s follow the steps to find the volume of the hemisphere if the radius is 3 cm.
Step 1: The radius of a hemisphere is 3 cm, which can be denoted as ‘r’.
Step 2: Substitute the value of the radius in the formula2πr3/3 and write the value of π as 3.14.
Step 3: Now calculate the value by 2 x 3.14 x 3x3x3/3 = 56.52 cubic units.
Also Read: Difference Between Power and Exponent
Solved Examples
- Find the volume of a hemisphere with a radius of 3 cm.
The volume of the hemisphere= 2πr3/3
= 2x 3.14 x (3)3/3
= 6.28 x 27/3
= 6.28 x 9
= 56.52 cubic units
- Find the volume of the hemisphere with a diameter of 12 cm.
Diameter of hemisphere= 12 cm
Radius of hemisphere= 12/2 cm
= 6 cm
The volume of the hemisphere= 2πr3/3
= 2x 3.14 x (6)3/3
= 6.28 x 216/3
= 6.28 x 72
= 452.16 cubic units
Related Posts
FAQs
A hemisphere is formed when a plane divides a sphere into two equal parts from its centre. So a hemisphere is half of a sphere. For example, the earth is a sphere divided into two hemispheres: northern and southern.
The volume of a hemisphere refers to the space occupied by a hemispherical object. Any object with a large volume takes up a large space. The volume of a hemisphere can also be described as half the volume of a sphere. This formula is calculated by Archimedes.
The volume of the hemisphere= 2πr3/3 cubic units.
Where, π = 3.14
r= radius of the hemisphere.
This was all about the “Volume of Hemisphere”. For more such informative blogs, check out our Maths Section, or you can learn more about us by visiting our Study Material Section page.