One app for all your study abroad needs
The perpendicular axis theorem majorly deals with the moment of inertia. The moment of inertia is a scientific term in physics that deals with rotating objects! The term explains to you how much resistance an object has to changing its spinning motion. The more spread out the mass is, the higher the moment of inertia and the harder it is to get it spinning or stop it from spinning. Have you ever thought about how a frisbee spins differently when you hold it at the flat level or by its edge? It all depends on the moment of inertia and if you want to analyse not just its spin on a flat surface, but along its edges, the perpendicular axis theorem formula is applied to know about it.
Table of Contents
- 1 What is the Perpendicular Axis Theorem?
- 2 Definition Of Perpendicular Axis Theorem
- 3 Perpendicular Axis Theorem Applications
- 4 How to Apply the Perpendicular Axis Theorem?
- 5 Difference Between Perpendicular Axis Theorem and Parallel Axis Theorem
- 6 Perpendicular Axis Theorem vs. Parallel Axis Theorem
- 7 Examples of Perpendicular Axis Theorem
- 8 Advantages And Limitations of Perpendicular Axis Theorem
What is the Perpendicular Axis Theorem?
The perpendicular axis theorem is a fundamental concept in the field of physics and engineering. It tells us more about the distribution of mass and moment of inertia of an object rotating around an axis.
- According to this theorem, the moment of inertia of an object about an axis perpendicular to its plane can be calculated by summing the moments of inertia of the object around two perpendicular axes lying in the plane of the object and intersecting at the perpendicular axis.
- In simple terms, the theorem says that the moment of inertia about an axis perpendicular to the plane of an object is equal to the sum of the moments of inertia about two mutually perpendicular axes in the plane of the object.
Also Read – Concept Of Parallel Axis Theorem
Definition Of Perpendicular Axis Theorem
The perpendicular axis theorem can be defined for a planar body (a flat object with negligible thickness), the moment of inertia about an axis perpendicular to its plane can be calculated by adding the moments of inertia about two perpendicular axes lying within the plane. All three axes (perpendicular and the two in-plane) must intersect at a common point.
Mathematically, the formula of Perpendicular Axis Theorem is –
Iz = Ix + Iy
Where:
- Iz = Moment of inertia about the perpendicular axis (like the record’s spin)
- Ix = Moment of inertia about an in-plane axis along one direction (like spinning the record flat in one direction)
- Iy = Moment of inertia about an in-plane axis along another direction perpendicular to the first one (like spinning the record flat in another direction).
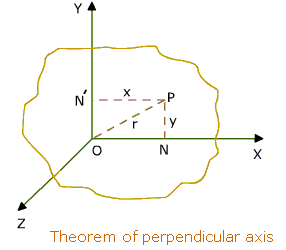
This formula explains that the moment of inertia perpendicular to the object (Iz) is simply the sum of the moments of inertia along two in-plane axes (Ix and Iy). This makes calculating the perpendicular moment of inertia much easier, especially for complex shapes.
Perpendicular Axis Theorem Applications
The perpendicular axis theorem finds its applications in the –
- Analysis of rigid bodies and rotational motion.
- Engineering and Physics to calculate the moment of inertia of complex shapes and structures.
- Determining the distribution of mass in rotating objects and predicting their rotational behaviour.
Also Read – – Algebraic Identities: Examples and Chart
How to Apply the Perpendicular Axis Theorem?
To apply the Perpendicular Axis Theorem, follow these steps:
- Identify the axis perpendicular to the plane of the object.
- Calculate the moment of inertia of the object about two mutually perpendicular axes in its plane using the appropriate formulas.
- Add the moments of inertia calculated in step 2 to obtain the moment of inertia about the axis perpendicular to the plane of the object.
Difference Between Perpendicular Axis Theorem and Parallel Axis Theorem
The difference between the Perpendicular Axis Theorem and the Parallel Axis Theorem is given in a tabular form –
Perpendicular Axis Theorem vs. Parallel Axis Theorem
| Feature | Perpendicular Axis Theorem | Parallel Axis Theorem |
| Axis of Rotation | Perpendicular to the plane of the object | Parallel to a known axis but shifted by a distance (d) |
| Object Type | Planar bodies (flat objects) | Any rigid object |
| Requires | Moments of inertia about two in-plane axes (Ix & Iy) | Moment of inertia about an axis passing through the center of mass (Ic) |
| Formula | Iz = Ix + Iy | I’ = Ic + Md^2 |
| Outcomes | Calculates the moment of inertia about an axis perpendicular to the plane | Calculates moment of inertia about a shifted axis |
| Limitations | This only applies to flat objects | Requires knowing the moment of inertia about the center of mass |
| Benefits | Simplifies calculations for complex shapes | Useful for analyzing off-center rotations |
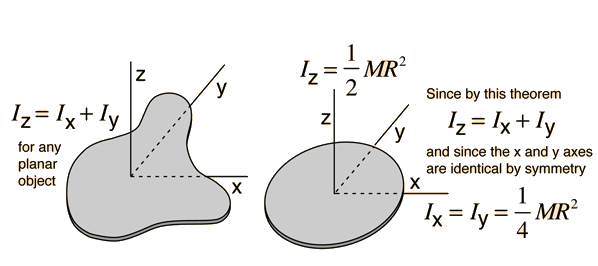
Examples of Perpendicular Axis Theorem
Imagine you’re trying to spin a pizza on your finger. How easy or hard it is to spin depends on two things:
- Mass of the pizza: A heavier pizza will obviously be harder to spin.
- Spread of the toppings: If the toppings are all clumped in the center, it’s easier to spin. But if the toppings are spread out all the way to the edges, it becomes more difficult.
This “spread-out-ness” of mass for spinning things is called the moment of inertia. If you know how easy it is to spin the plate flat in two directions (like along its length and width), you can figure out how hard it is to spin it upright. It does this by adding the moments of inertia for the flat spins (around the in-plane axes) to find the moment of inertia for the perpendicular spin (around the vertical axis).
Let us understand through another example of a Disc!
Advantages And Limitations of Perpendicular Axis Theorem
The Advantages of the perpendicular axis theorem are –
- Effortless Calculations: This theorem allows to find the complex perpendicular moment of inertia (Iz) by simply adding the easier-to-calculate in-plane moments of inertia (Ix and Iy).
- Works for Many Shapes: It applies to various flat shapes and various geometric shapes and configurations.
Limitations of the perpendicular axis theorem are –
- Flat Objects Only: The theorem only works for flat objects like discs, rectangles, or triangles. It cannot handle 3D shapes like balls or toys.
- Common Meeting Point: All three axes (perpendicular and the two in-plane) must intersect at a single point. Otherwise, the theorem is not proved.
Related Blogs
Hope this blog helps you. Keep reading more of our blogs to learn about the basic concepts of Physics!