One app for all your study abroad needs
If you are here, it means you want to know in simple terms what the Parallel Axis Theorem is and how it applies to the world of physics. Do not worry, we are here to help! In this article, we will try to understand the basic concept of parallel axis theory, explain the parallel axis theorem formula along with the details of this fundamental principle and break it down into easy-to-understand points. So, without further ado, let us jump right into the world of Physics!
Table of Contents
History of Parallel Axis Theorem
The theorem is believed to have originated in the 16th century by polymath Christiaan Huygens when he introduced the concept of moment of inertia for the first time during his research on the compound pendulum and wrote in his work “Horologium Oscillatorium” (1673).
Later, a Swiss mathematician, Jakob Steiner also added an important geometric configuration factor to the theorem. Thus, this theorem also came to be known as the Huygens- Steiner theorem.
What is the Parallel Axis Theorem?
The parallel axis theorem is a fundamental principle in physics and engineering that helps in calculating the moment of inertia of an object that is not centered on its mass distribution axis. Now what is a moment of inertia, it is a measure of an object’s tendency to resist angular or rotational motion. The larger the moment of inertia of an object, the more force is required to make it rotate.
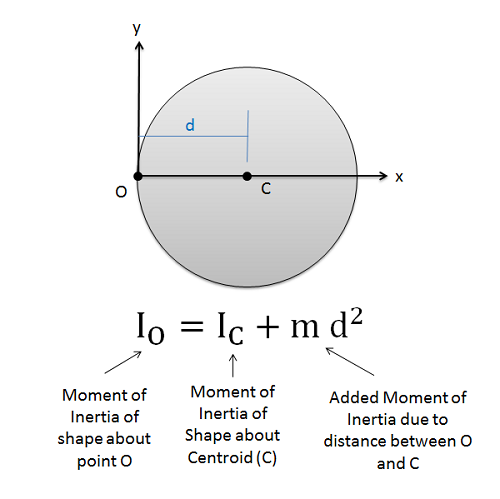
- Parallel Axis theorem, establishes a relationship between the moment of inertia of a rigid body about an arbitrary axis and its moment of inertia about a parallel axis passing through the center of mass.
- This theorem simplifies the calculation of moments of inertia for various axes, making it a valuable tool for engineers, physicists and anyone studying rotational motion.
Also Read – Gauss Elimination Method: Steps, Examples and More!
Definition Of Parallel Axis Theory
The theorem of parallel axis states that the moment of inertia of an object around an axis that is parallel to its center of mass is equal to the sum of the moment of inertia about the center of mass and the multiplication of the object’s mass and the distance between the two axes squared.
- Mathematically, the formula for the parallel axis theorem can be written as –
I = I_cm + Md^2
where:
- I = Moment of inertia about the desired axis (not necessarily the center of mass)
- Icm = Moment of inertia about an axis parallel to the desired axis but passing through the center of mass
- M = Total mass of the body
- d = Distance between the two parallel axes(the desired axis and the axis through the center of mass).
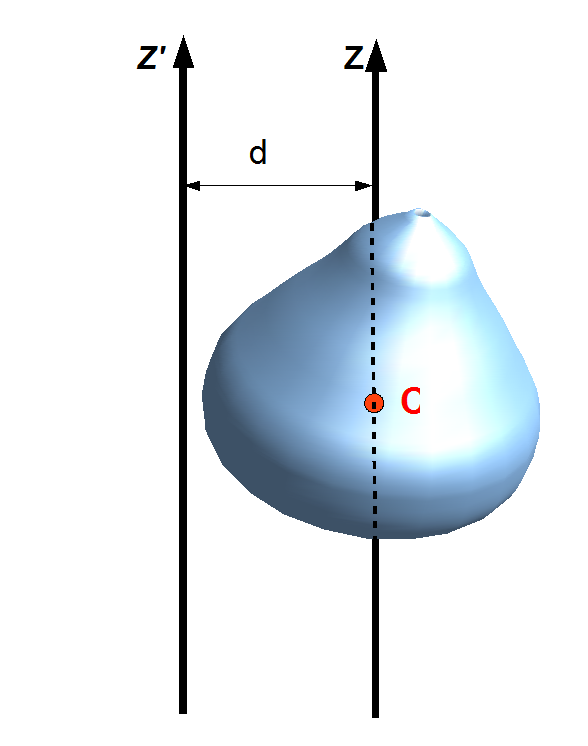
Here’s a breakdown of what the theorem says:
- Moment of inertia (I): This represents an object’s resistance to rotational acceleration.
- Parallel axes: These are imaginary lines that run parallel to each other.
- Center of mass: This is the point where the entire mass of the object is considered to be concentrated.
The theorem states that the moment of inertia of an object about an axis (let’s call it axis A) parallel to another axis passing through its center of mass (axis B) can be calculated by adding two terms:
- The moment of inertia of the object about the axis going through the center of mass (I_cm).
- The product of the object’s total mass (M) and the square of the distance (d) between the two parallel axes (A and B).
Parallel Axis Theorem Application
The parallel axis theorem is used in engineering and physics to calculate the moment of inertia of complex objects.
- It is especially useful when dealing with irregularly shaped objects or objects with varying density distributions.
- By applying the parallel axis theorem, engineers and designers can accurately determine the inertia of components in mechanical systems, which is essential for analyzing their stability and response to external forces.
Also Read – Algebraic Identities: Examples and Chart
How to Apply the Parallel Axis Theorem?
- To apply the parallel axis theorem, first, calculate the moment of inertia of the object about its centre of mass using standard formulas or methods.
- Next, determine the distance between the center of mass and the parallel axis of rotation.
- Finally, use the parallel axis theorem formula to calculate the moment of inertia about the parallel axis.
Example of the Parallel Axis Theorem in Action
- Let’s consider a thin rod of length L and mass M rotating about an axis perpendicular to its length and passing through one end.
- The moment of inertia of the rod about its center of mass (which is at L/2) is given by I_cm = ML^2/12.
- If we want to find the moment of inertia of the object i.e. rod which is about an axis parallel to its center of mass and passing through the other end, we can use the theorem of parallel axis.
- The distance between the two axes is L, so the moment of inertia about the parallel axis is given by I = ML^2/12 + M(L)^2 = ML^2/3.
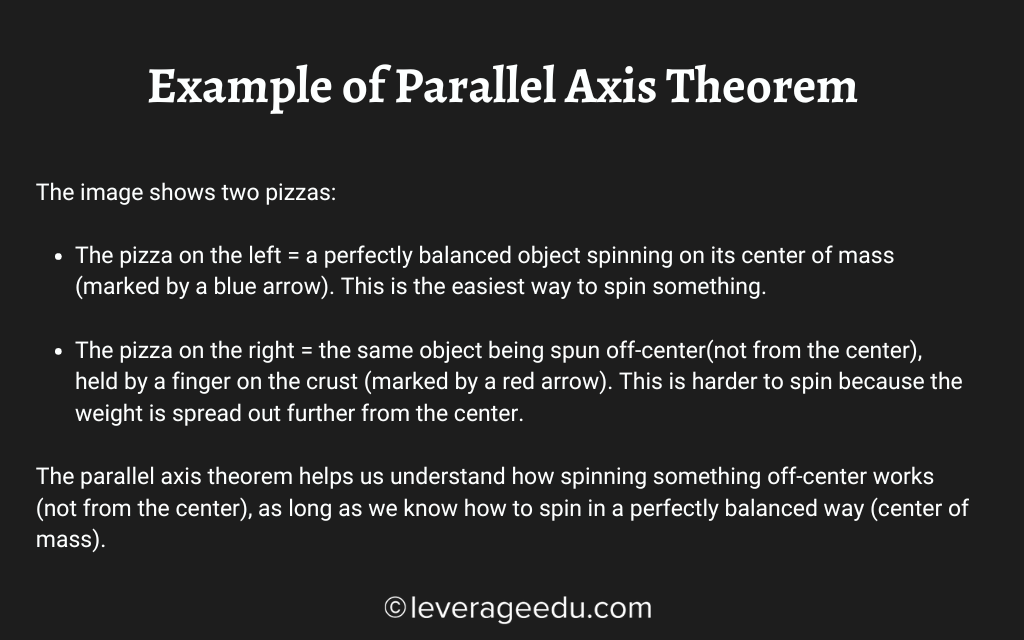
Let us understand through an example of Pizza!!
Advantages and Limitations Of Parallel Axis Theorem
The advantages of the theorem are –
- When calculating the moments of inertia around any axis, it’s often easier to determine the known moment of inertia about the center of mass first. This method allows for the calculation of moments of inertia around any axis.
- This theorem can also be used for various hard objects, no matter their shape or size.
However, here are some of the limitations of the theorem –
- This principle holds true only when the axes are parallel and cannot be applied to non-parallel axes.
- The object being analyzed must be a rigid body, which means that it must retain its shape during rotation and not change its form.
Related Blogs
Hope this blog helps you. Keep reading more of our blogs to learn about the basic concepts of Physics!